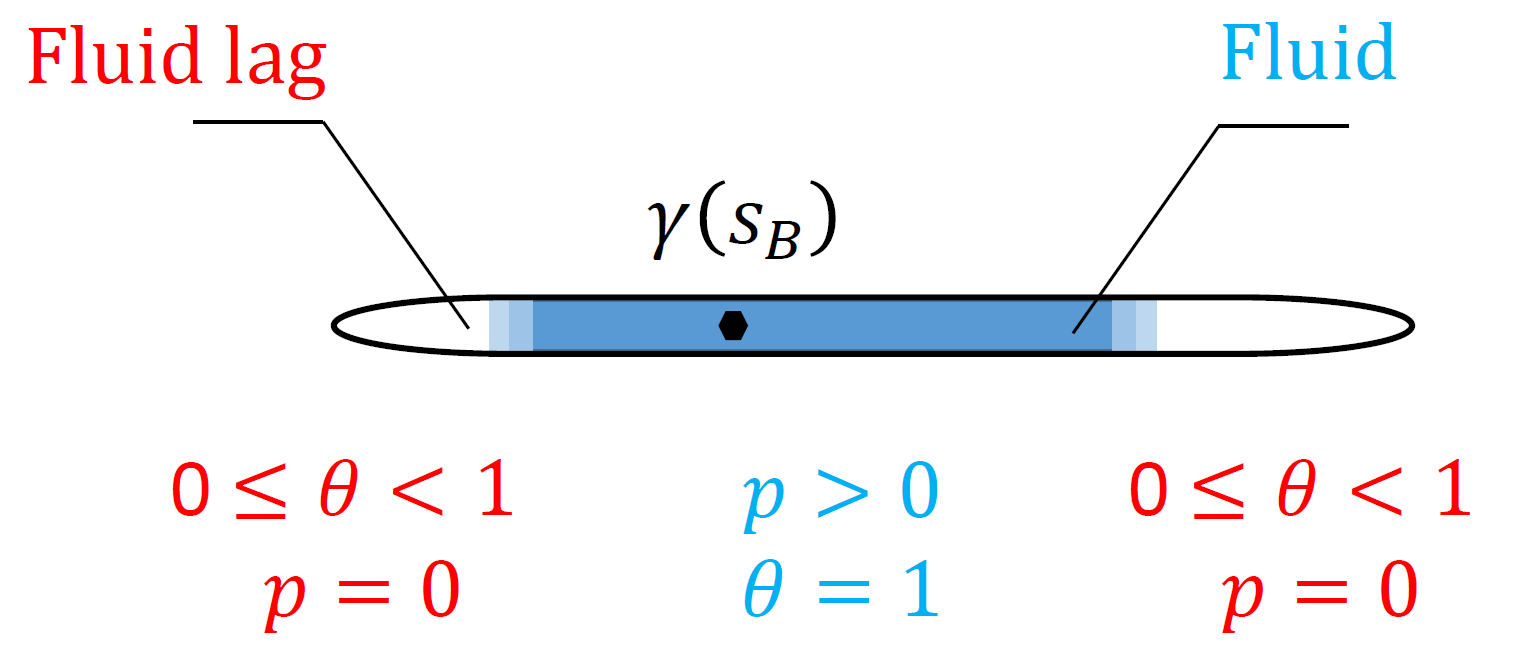
An efficient method to model the fluid lag in hydraulic fracturing has been developed based on the Elrod-Adams model. The main feature of this method is the absence of the need to explicitly track the free end of the fracturing fluid, but rather,the fluid front is obtained by solving the pressure field (zero for the lag) and an auxiliary field for the entire fracture.

The main idea of the Elrod-Adams model is to introduce a liquid fraction field θ: Γ →[0,1], whose physical meaning is the percentage of liquid occupying the fracture at s$ More precisely, θ(s)=1 means that liquid is completely filled at s, while the region with θ(s)=0 corresponds to the part of the fracture with no fluid. Correspondingly, the pressure p(s) is positive only when θ(s)=1 (Figure 1).

With these definitions, the governing equations of the liquid inside the fracture reads

Equation (1a) is the main equation of the Elrod-Adams model, which is merely a restatement of mass conservation. Equation (1b) describes the condition for the pressurized zone, while  (1c) both the transition zone {0< θ <1} and the dry zone {θ=0}. Equation (1d) enforces  mass-conservation at the fluid front boundary.Equation eqref{EAFlowRate} models the flow injection as a discontinuity in the flow rate, and Equation (1e) is the condition for the withdrawal stage.

An important advantage of this method is that no change of formulation, and hence no contact detection, is needed when the fluid reaches the fracture tip. Moreover, the method works for both the injection phase and the liquid withdrawal phase. Based on the latter case studies can be developed to investigate the quantity of the remaining fluid after the fracturing process in order to assess the environmental impact of fracturing. The method applies to both 2D and 3D problems.

Fig1: The essence of the Elrod-Adams model is to employ a liquid fraction field θ defined over the entire fracture Γ that assumes value between zero and unity. It represents the percentage of liquid occupying the fracture width at s. In particular, θ(s)=0 means that there is no liquid at s (the dry zone), while θ(s)=1 means that the liquid is completely filled at s.